Making (Number) Sense

This document lives at mrfloresreads.info/resources/math/making_number_sense.html

Introduction
How Can You Do It?
- General Tips
- Activities
  - Prenumber Activities
  - Number activities
BIBLIOGRAPHY

Introduction

Number sense is a foundational understanding of how numbers work. Researchers have demonstrated that every child can develop number sense. Unfortunately, it has also been found that many children don’t. This is because number sense does not develop by accident. It is not a “side effect” of doing puzzles, songs, or other informal activities that may be superficially related to math. Teachers are increasingly working to build number sense, especially in the early grades. However, you can give your children a jump-start by developing number sense before they start school, or if they are already in school, you can help them strengthen their number sense to be better prepared for deep mathematical understanding and success.

How Can You Do It?

General Tips

Explaining
Encourage your child to explain his reasoning. This not only gives you insight into how he thinks, but it also helps the child evaluate his thinking and “cement” it in place.

Be sure to ask your child to explain her thinking all the time, not just when she makes a mistake. Constantly asking sends several important messages: your ideas are valued; math is about reasoning; and there are always other ways to look at a problem.

Real-world links
As much as possible, link math to real-world situations. When shopping, compare prices. When walking around the neighborhood or in the park, estimate distances using steps and/or standard measurement (feet, miles). Allow your children to pay at the checkout and count their change to make sure it is correct (matching the receipt, not necessarily doing a subtraction problem).

Measurement
When teaching your child mathematics, make her verify estimates through doing. Point out that measuring is important, even though it is always an approximation since it can always be made more precise. For example, a pencil that looks 5 inches long might be 4 3/4 using a ruler with 1/4 inch marks or 4 11/16 on a ruler with 1/16 marks.

Numbers versus numerals
As you work with your child, remember that “number” is the amount of the things you are counting or measuring. The “numeral” is the shape we have decided to use to record it. For example, five beans on a plate can also be represented as five fingers on a hand, the oral or written word “five”, five tally marks on a page, a drawing of five beans, or the numeral 5. Connecting the glyph “5” to the amount of beans on the plate should be the last thing to focus on. It is far more useful for children to understand that five can be represented all those other ways.

Activities

These activities are divided into two types, prenumber activities and number activities. Prenumber activities are important for developing concepts that support and eventually lead to understanding the concept of numbers. They also help in developing understanding of problem situations (problem solving and critical thinking) and so should not be ignored.

You can do activities for as long or short a time as is comfortable (you will be there working along with your child). But you should be sure to do the activities multiple times over the course of several days. Later on, revisit activities that you haven’t done together in a while.

Prenumber Activities

Classifying

Button Box Sort
It’s called “Button Sort” but any objects can be used for this activity. Give your child a set of objects to sort based on some attribute. Objects that work well include: buttons; leaves; coins; photographs; silverware; books; socks; etc. You might also give him a small amount of mixed dry beans to sort. Another benefit of this activity is that your child will also be developing small motor skills in finger manipulation.
– EXTENSION - After sorting objects one way, challenge your child to sort them a different way. For example, after sorting the buttons by size, have her sort them by color or the number of holes.

Patterning

Making Patterns
Again using small objects, begin a pattern and have your child continue the pattern. For this activity, you could use different color/sized beans, buttons, coins, etc. The child could also use crayons, markers, or colored pencils to continue a coloring pattern on a page.
– TIPS - Begin with simple patterns, for example ABABAB, then move on to more complex or longer patterns like ABCBABCB.
– Start with linear patterns (the pattern goes in a line) then move on to two-dimensional patterns (rows and columns). A two dimensional pattern might look like this:

✿✿  ✿✿  ✿✿
  ✿✿  ✿✿  ✿✿
✿✿  ✿✿  ✿✿

– Include transformation patterns as the child gets more skilled at continuing patterns. Transformation patterns are those where the pattern changes by movement instead of color, shape, etc. For example, using toothpicks:

— | — | — | — | — | — | — | — |

Comparing

Questioning
To practice the skill of comparing, simply ask your child questions that can be answered with descriptive responses like “more than”, “less than”, “as many as”, “the same as”, or “is equal to”. The Button Box Sort activity lends itself well to these types of questions, but so do everyday events:
– Are there more boys than girls in your class?
– Do we have enough plates for everyone in our family to eat dinner?
– I have 3 dimes and 3 nickels, is that equal? (This one will be challenging!)

Conservation

Using everyday objects and situations, provide opportunities for your child to discover that change the size or organization of objects does not change the quantity of objects. (Don’t worry, you don’t have to teach your child the words “organization”, “conservation”, or “quantity”!)

Spread out
After showing your child 5 objects (pennies, gummi bears, etc.) all grouped together, count them. Then spread the objects apart and ask your child if there are more now. After responding, your child should count them again to see it is the same.
Two groups
Make two groups of jellybeans, the same number in both groups. In one group, have the jellybeans all squished together, in the other have them spread apart. After guessing which has more, have your child count each group, or have him line up the jellybeans from one group next to a line of the jellybeans from the other group.

Group Recognition (Subitizing)

The activities used to develop this skill may take a little longer to prepare, but do not skip them! This skill is highly correlated to better understanding and scores in math.

How Many?
Show your child a small set of objects for a few seconds and then hide them. Ask them “How many?” and have them guess then count to check their guess. Most kindergartners and first graders will be able to instantly tell how many there are in sets of up to 5 or 6 objects.

TIPS

Change the ways the objects are arranged. For example, one time you might show 5 beans scattered at random (hardest to subitize), lined up in a row (easiest for kids up to about 5 or 6 years old), or in a square pattern like on dice (easiest for 2nd grade on up).
Use a paper plate to hide the objects while you arrange them, then “flash” them to your child for 1/2 second up to 2 seconds depending on your child’s development and the number of objects.
Keep the numbers between 1 and 6 at first, using either single groups or two groups (for example, a group of 2 and a square of 4 to make 6). If the child is ready, you can move on to larger numbers (between 6 and 10), again using groups of 5 or less (for example three rows of three to make 9).

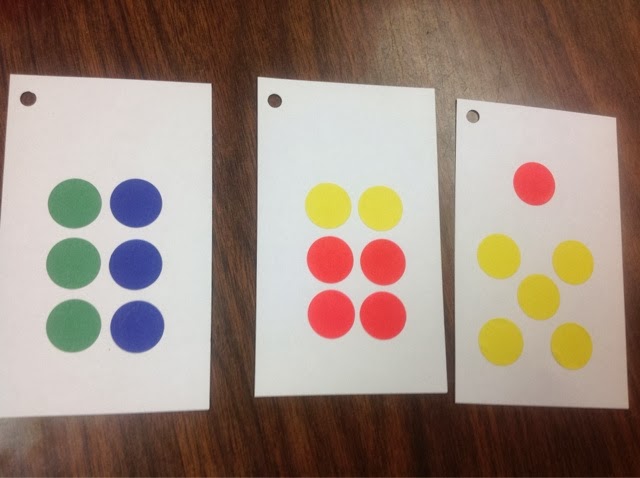
Make sets of cards for the activity for quick review or to play “on the road”. Just use a marker or stickers on index cards and make a set of cards with lots of different variations.
– Use a single color for each “set”. If the card is showing a “4” like : : , keep all dots the same color. But if you have a card with 5 made from a set of . . . and : , the three dots could be one color and the two could be another color if you want.
– If you want a complete set of cards, you can get them from math.mpls.k12.mn.us/uploads/subitizing.pdf to find multiple printable sheets of subitizing cards including more info and games!
On Mr. Flores’s website, there is an online version of this activity at mrfloresreads.info/resources/math/dailymath/dots.html . If you decide to use this, be sure to still work with your child; do not have them work on it independently.

Number activities

Counting

Cardinality
This is a super simple activity that must not be overlooked! Hand your child a bunch of items (within her counting range) and ask her, “Count these, please.” After she counts them, ask her, “How many are there?”. She should be able to simply tell you the amount. Younger children and children who have not developed the understanding of cardinality will count them again. If this is the case, let her count them again, then reassure her, “That’s right, there are __ things.” Repeat this activity over a number of weeks until she starts to see that every time she counts, the last number she says is the amount you verify as the “right” number. Cardinality is generally well established by the time a student enters kindergarten but some children may take longer.

BEFORE & AFTER Children often have difficulty with the concepts of "before" and "after" in numbers as well as events. These concepts must be developed over time through numerous experiences. Discuss before and after events as well as asking about numbers. For example, "What did Pooh do before he went to see Tigger?"

Counting out loud
Counting includes counting by rote out loud, counting objects, and skip counting by various amounts.
– Practice counting out loud from 1-20 whenever there’s a chance. Your child should be fluent in counting to 20 before attempting to count objects.
– After reaching fluency to 20, have them try “counting on” from a number besides one. Also, occasionally ask them what number comes after a given number. We want them to be able to do both of these without having to start from one each time.

– Practice counting out loud backwards, initially from 10 then later count backward from 20. After they are fluent with these, ask them to count backward from other numbers. Finally, ask them to tell you what number comes before a given number.
– Count larger numbers by rote starting from a multiple of ten, for example 40 or 70. Point out that there is a pattern when counting, that all they need to do is say the ten and then count by ones, for example “40, 1, 40, 2, 40, 3 …” for counting 41, 42, 43.
– Practice skip counting by 10s. After reaching fluency starting with 10, have your child start at a different ten, for example start at 40. Don’t forget to also count backwards!
– After reaching fluency with 10s, practice counting by 10s starting at any number other than a multiple of 10. Instead of just counting “10, 20, 30 …”, start at 27: “27, 37, 47, 57”.

Once your child is fluent with counting by ones to 20, you can begin doing the following activities with them. Don’t forget to continue oral counting frequently!

Counting objects
Give your child a set of objects. Let him count the objects using whatever technique or strategy he chooses. Any objects around the house would work well for this activity (beans, pasta, cereal, socks).
Counting coins
Give your child a set of coins and have her count the total amount. Begin with pennies (counting by ones) before moving on to groups of other coins (for example, a bunch of nickels for skip counting by 5s – 5, 10, 15, 20). Once she can count all the coins in single groups, begin mixing coins, for example, nickels and pennies together. Eventually, we would expect her to be able to count a bunch of mixed coins.
– You could make a game out of putting the coins in your pocket at the end of the day on the table. If your child can correctly count them allow her to put the coins in a could put them in the bank. They could then periodically count the bank to see how much they have saved so far.
Calendar or Counting Chart
In class teachers use a tool called a “hundreds chart”. Most families do not have one in their homes already, but a quick Google image search provides numerous examples. Guruparents.com/hundreds-chart.html has more info and two colorful versions that can be printed out, including Mr. Flores's preferred 0-99 upside-down version^*. Families can download or make their own hundreds chart or simply use a calendar since many of the activities can be done on either.
– Pick a day. Ask the child to count forward or backward from that day.
– Look for patterns. What do you notice about all the numbers in a given column?
– Cover up a square. Ask your child what number is covered up and how they know.
* Mr. Flores prefers his own “upside-down” hundreds chart, mrfloresreads.info/resources/math/upsidedown0-99.pdf as it conceptually emphasizes that “bigger” numbers are “higher” and counting numbers go “up”.
Skip counting
– Practice skip counting by 2s and 5s. Ask your child to listen for patterns as they count. For 2s, they probably won’t hear any patterns until they get to numbers higher than 20; but don’t go there until they are really solid with counting by 2s to ten. You can also print out a hundreds chart (see above) and have your child use a crayon or highlighter to color the numbers as they count. This will visually reveal the counting pattern.
– For extra challenge, use a calculator to practice skip counting by other numbers. For example, to count by 4s, punch in “0 + 4 =”. Then if you push “+4=” again each time, it will skip count by 4s (some calculators will do this if you simply hit “=” over and over). You can even use the subtraction key to skip count backwards. As you play around with the calculator together, ask your child to predict what the next number will be before you hit the “=” button.

Odd and Even Numbers

Using numbers up to 20, count out some items. Have your child arrange them in pairs. Each pair is even, so if you have no items left over, the whole number is even. If there is one left over, then the number is odd.
Instead of grouping them in pairs, make two lines next to each other, making sure each item in one line is directly across an item in the other. If all the items are “partnered” then the number is even; if there is one item without a partner, the number is odd.
Another way of determining whether a number is odd or even is to “share”. Take an amount of items and share them between your child and yourself, doing so in a “One for you, one for me” pattern. After dividing up the items, you each count your items. If they are the same amount, the number is even; if one of you has more, the number is odd. Have your child repeat the activity with another number. (Remind him that for finding out whether a number is even or odd, he will only be sharing between two people, himself and one other person.)
After doing these activities, ask your child to notice any patterns. For example, they may remember that 3 is odd and so is 13. When this happens, try other numbers like 23 to see if they fit the pattern. Eventually, you and your child should be able to generalize that only the “ones” digit determines odd or even numbers since higher numbers are just groups of ten and ten is always even.
After your child has had many experiences with figuring out odd and even numbers, start asking him to predict whether a number is even or odd before you find out together.

Estimation

Good estimation skills develop slowly. As you work on these activities, focus on having your child get “close enough” rather than exact answers. Have discussions when the estimates are not reasonable; explain that “reasonable” estimates have good reasons behind the number, for example we do not need to buy 50 Valentine cards because there are only four students at each table and 8 tables of four is a lot less than 50. Keep the number of objects used for estimating within a range of numbers familiar to your child. For many new first graders, numbers within the range of 10-30 are appropriate. When estimating and counting larger numbers, have your child put the items into groups of “about 10” to count by tens to find a number close to the actual amount.

Guess How Many
Have your child questions like the following. After guessing, have your child count to find the actual amount. Praise her for getting close enough. Discuss guesses that might be unreasonable.
– How many M&Ms in a bag?
– How many Cheerios can fit on a spoon?
– How many grapes are in the bowl?
– How many cookies will fit in the container?
– How many people in the store? (Show her how to “cluster” people in groups of 5 or 10 to make estimating easier.)
– How many cars are in the parking lot?
– How many days until __? (This may lead to a discussion of approximate days in a month in order to give a reasonable estimate.)
– How many seconds will it take to run around the house? (Challenging! Children often have a very poor feel for the passage of time.)
– How many steps? (While walking with your child, identify an object up ahead a short distance away. Have her guess how many steps it might take her to walk there. Then count her steps as you walk to the object. BONUS CHALLENGE: using regular sized steps, who will need more steps to reach it? About how many more?)

Mental Math

Real life often requires mental computation. Our children need to be able to move numbers around in their heads and discuss their strategies. They also need to be able to do it fluently, though not necessarily “fast”. For these activities, you will be saying an extended sequence of operations to your child, one part at a time. Pause after each operation to give your child a chance to perform the operation in his head.

EXAMPLE: “Start with 4, (pause) add 3, (pause), add 2 more, (pause) then add 3 again. How much is that? (12) ”

After hearing the answer, ask your child how he got the answer. He may repeat the sequence to you and explain his answers along the way, or he may explain how he manipulated the numbers. For example, “You said to add 4, 3, 2, and 3. I know that 3 and 3 are 6, and 4 plus 2 is 6. So I have two 6s. 6 plus 6 is 12!” The manner of arriving at the answer is not so important as being able to recall/explain how.
TIPS
- Start with a sequence using 3 numbers. Gradually move up to longer sequences as your child gains proficiency.
- Use smaller numbers (1-5) first. Use single operations to begin with (only adding or only subtracting). Combine operations later as your child shows fluency and accuracy in single operations.
- Include adding and subtracting 10, but avoid numbers higher than 10 unless/until your child is very good at adding and subtracting 2-digit numbers without confusing the tens and ones.
- You can start simply by using “plus” and “minus” or “take away”. Later, use “add” and “subtract”. Don’t be afraid to use “increase it by” and “decrease it by” later on!

Number Sequencing (number ordering)

Write Them
Cut five index cards in half crosswise to get ten small cards. Have your child write a range of numbers, one on each card. For example, have her write the numbers from 30 to 39. Do this many times over the course of many days, saving the cards to use for the following activities.
Sequencing
Give your child a range of ten numbers from the sets she has written (during the Write Them activity). The cards should include all the numbers within the range, for example all the numbers from 50 to 59. She should mix them up (shuffle them) and then lay them out on the table them into the correct order. Make a game out of it to see how fast she can do it.
– Begin by only including numbers within the same group of tens, for example only thirties or only teens.
– Later include ranges that cross a ten, for example 65 to 74.
– Once she is good at sequencing a complete range, start giving her sets of ten random cards.

Adding and Subtracting

Be sure to start with composing and decomposing activities. Composing is just putting numbers together to make new number. Decomposing, then, is taking a number and breaking it into two or more smaller numbers. Doing these activities with counters for a long time before introducing written number sentences (equations like “4 + 5 = ”) builds an understanding of how numbers work. Putting numbers together results in a larger number. A number can be broken into many different combinations of numbers that all make the same amount when recombined.

To do these activities, use counters like dry beans. (Beans work well because they are hard and cheap, but you can use beads, buttons, or any other small objects, though I would stay away from cereals for these activities.)

Composing
Give your child two small piles of counters. Have him count each pile separately, then count them all together. Come up with a sentence that explains the amount, for example, “Four and five together make nine”. You can also use terms like “add” and “plus”. Do not worry about writing anything down! This is all about building number understanding, not about “doing math”.
Decomposing
Give your child a small pile of counters. Ask him to count the pile. Then pull (or have him pull) a smaller pile away from the original pile. Don't put it back into the bag of beans, just pull it a little ways away from the original pile. Have him count how many were pulled away, then count how many were left in the original pile. Say a sentence to explain the amount, like, “Thirteen take away four leaves nine.” You can use terms like “minus”, but again, this is not about “doing math”, so don't bother writing it down.

Story Problems
Tell your child a simple story problem. Allow her access to counters and allow her to figure it out on her own. Resist the urge to “show her how”! Let her play with the counters to come up with a solution. If she does not even know how to begin, point out that the beans can represent the things in the story. Tell the story again, using the counters as props. Show how the counters can lead to the solution.
- Keep the stories simple, especially at first. Example, “Five boys and 6 girls were playing soccer. How many kids were playing soccer?”
- As your child gets stronger at solving story problems, introduce more complex types. In the following table, the top left corner is the easiest, and the bottom right corner is the most difficult.

	Result unknown	Change unknown	Start unknown
Joining	Grandmother had 5 strawberries. Grandfather gave her 8 more strawberries. How many strawberries does Grandmother have now?	Grandmother had 5 strawberries. Grandfather gave her some more. Then Grandmother had 13 strawberries. How many strawberries did Grandfather give Grandmother?	Grandmother had some strawberries, Grandfather gave her 8 more. Then she had 13 strawberries. How many strawberries did Grandmother have before Grandfather gave her any?
Part-Part-Whole	Grandmother has 5 big strawberries and 8 small strawberries. How many strawberries does Grandmother have altogether?	Grandmother has 13 strawberries. Five are big and the rest are small. How many small strawberries does Grandmother have?
Separate	Grandfather had 13 strawberries. He gave 5 strawberries to Grandmother. How many strawberries does Grandfather have left?	Grandfather had 13 strawberries. He gave some to Grandmother. Now he has 5 strawberries left. How many strawberries did Grandfather give Grandmother?	Grandfather had some strawberries. He gave 5 to Grandmother. Now he has 8 strawberries left. How many strawberries did Grandfather have before he gave any to Grandmother?
Compare	Grandfather has 8 strawberries. Grandmother has 5 strawberries. How many more berries does Grandfather have than Grandmother?	Grandmother has 5 strawberries. Grandfather has 3 more strawberries than Grandmother. How many strawberries does Grandfather have?	Grandfather has 8 strawberries. He has 3 more strawberries than Grandmother. How many strawberries does Grandmother have?
Multiplication & Division	Grandmother has 4 piles of strawberries. There are 3 strawberries in each pile. How many strawberries does Grandmother have?	Grandmother had 12 strawberries. She gave them to some children. She gave each child 3 strawberries. How many children were given strawberries?	Grandfather has 12 strawberries. He wants to give them to 3 children. If he gives the same number of strawberries to each child, how many strawberries will each child get?

Problem chart based on Cognitively Guided Instruction Problem Types (Carpenter et al., 1996)
- For ideas of stories to use, visit mrfloresreads.info/resources/math/dailymath/cgiprobs.html .

Base Ten Manipulation

When students trade with base ten manipulatives, they can demonstrate that the number 14 may be represented as 14 ones or as 1 ten and 4 ones. Being able to understand this concept of place-value is essential for understanding higher math concepts.

Making Tens
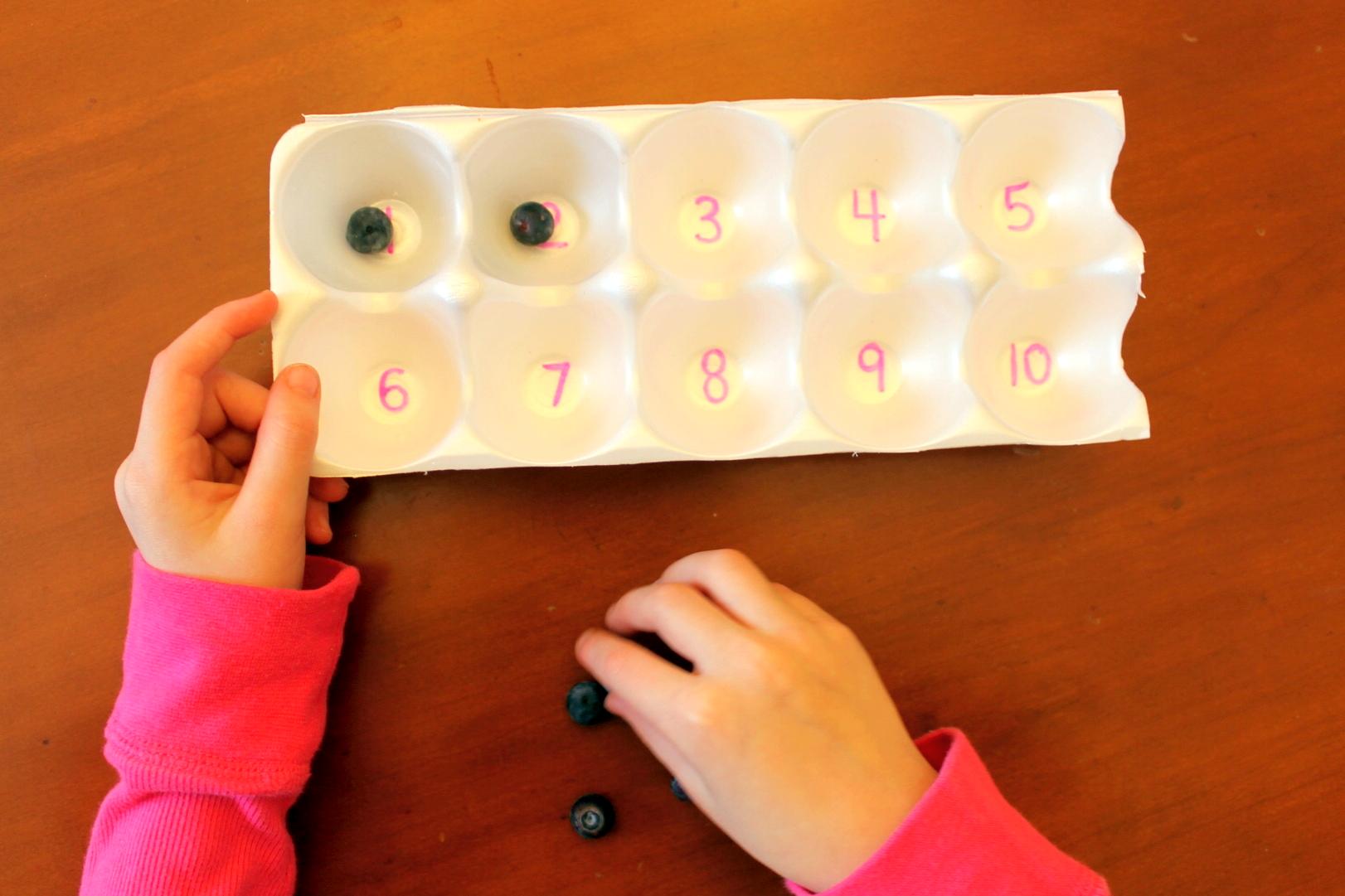
Make a simple “ten-frame” by cutting the top and two end cups off a one dozen egg container. You can write the numerals from 1 to 10 in the cups, but it is not necessary. What is important is that the child sees this as a group of ten. Give the child a random amount (9 or fewer) of cubes, coins, beans, etc. and have her count them. Then ask how many more are needed to make ten. Check by putting one object into each of the cups (filling the top row first) then counting the empty cups.
- Remember to always fill the top row before putting any objects into the second row. This will reinforce the concept of a frame of ten, which will be useful as the child moves to drawing amounts instead of using counters. In the following example, set A is easier to identify as a 6 needing 4 to make 10 because of the "spaces", while set B is less obvious because it is hard to know how far the "frame" extends.
```
A * * * * *    B  * * *
  *               * * *
```
- Encourage your child to count objects and empty cups from 5 instead of from 1 when amounts span a full row or more. For example, set A above should be counted, "Five, six", starting with the whole first row.
Grouping Tens
Give your child about twenty dry beans or other counters. Ask her to count them and tell you how many. Then ask her to “show” you. You are trying to get her to show her amount as a set of grouped beans. For example, if she has 24 beans, she should be able to make 2 groups of tens and 4 loose beans. She may also choose to make four groups of 5 and four left over or even 12 groups of 2 which she counts by skip counting. Do this a few times with increasing amounts of beans, pointing out that groups of ten are really easy to count. Hopefully, she will see that grouping by tens is efficient and useful. We want her to get to the point where she groups by tens automatically.

Trading Tens
As an extension of the Making Tens activity, have your child glue ten beans onto a popsicle stick (craft stick). After these “tens sticks” (“tens rods”) have dried, use them for this activity. Give him a bunch of beans (more than ten) and have him group them by tens as before. Point out that even though he has them grouped by tens, he actually still has 13 loose beans. Have him trade the group of ten loose beans for a “tens stick”. Now show him that he has 1 ten (write the digit “1”) and 3 loose ones (write the digit “3” next to the “1” to make “13”). Do this each time with numbers of various sizes. Make sure he groups the loose beans into groups of ten before trading them out for a “tens stick”.
– Watch that he does not simply grab a tens stick before he removes the group of ten loose ones. The total number of beans should not change.
– After he is good at this, challenge him to get the number of beans you ask him to get. at first he may simply count out 25 loose beans. If he does this, remind him to group them in tens and trade out the groups for tens sticks. He will soon be making the numbers you ask quickly by starting with tens sticks and counting out the ones after.
– Once he is proficient at these activities, ask him if there are other ways to make a number by trading in or out tens sticks for groups of ones. For example, what is another way to make 25 besides 2 tens and 5 ones. He could also make 25 with 1 ten and 15 ones, or he could make it with 25 ones. Each of these represents the amount 25!

Conclusion

These are just a few ways to develop number sense in your child. By working on these fun, simple activities with your child, you will be building important concepts and skills in number sense that are crucial for your child’s mathematics success. And remember to enjoy your time together!

If you are interested in reading more about number sense, contact your child’s teacher or read this document online at mrfloresreads.info/resources/math/making_number_sense.html.

What is Number Sense?

Number sense, simply speaking, is an understanding of the relationships between real-world quantities, the counting numbers in the student's spoken language, and the glyphs (numerals) and symbols used to represent them. (Griffin, 2004)

Number sense develops gradually in children. It comes about as a result of exploring numbers/quantities, playing with them, and paying attention to the ways numbers/quantities relate to other numbers/quantities. As number sense develops, children develop flexibility in thinking about numbers including the ability to represent numbers in multiple ways. They also develop an understanding of number magnitude (size) and are able to predict the effects of operations on numbers (e.g. adding positive numbers together results in a larger number).

Number sense can develop in some children through careful observation and play beginning at a very young age. However, as mentioned above, it does not just "happen" as a side effect. Many children need specifically guided instruction or participation in particular activities to develop number sense.

Why is it important?

Number sense enables students to understand and express quantities in their world. For example, whole numbers describe the number of students in a class or the number of days until a special event. Decimal quantities relate to money or metric measures, fractional amounts describing ingredient measures or time increments, negative quantities conveying temperatures below zero or depths below sea level, or percent amounts describing test scores or sale prices.

The development of number sense in students is important for many reasons. As well as those listed below, number sense is paramount in providing understanding not only of how numbers work together but also of why we manipulate them the way we do, i.e. why the algorithms work.

Number sense is the basis for understanding any mathematical operation and being able to estimate and make a meaningful interpretation of its result. Getting the right answer is important, but so is understanding why it is the right answer. In a study by Jordan, Locuniak, Ramineni and Kaplan entitled “Predicting First-Grade Math Achievement” from Developmental Number Sense Trajectories, it was shown that “early sense of numbers (sic) is a reliable and powerful predictor of math achievement at the end of first grade.” This, of course, predicts further success as the student advances through the grade levels, provided they continue to develop and implement their number sense.

Knowing the why of how numbers work is of utmost importance, and children should not be shown or encouraged to discover the “how” until they understand the “why”. Techniques such as using ten frames to represent numbers and using concrete models to show place value concepts are daily necessities for young children. Inquiry-based approaches (such as math dice games) to teaching children mathematics should be utilized as primary teaching methods over paper-pencil practice using numerals and symbols. This is not to say that explicit teaching number skills is not essential, especially for those students from low socio-economic status. We absolutely need to do this. It is saying that teachers should provide multiple opportunities for students to experience numbers and make connections before putting the pencil to paper.

Components of number sense

Number sense begins very early and must be a focus of primary grades math instruction. This is the solid foundation in math that all kids need. A sense of numbers is critical for primary students to develop math problem solving skills. The National Council of Teachers of Mathematics increasingly calls for districts to give more attention to building this skill, and studies have found that number sense accounts for 66% of the variance in first grade math achievement. The National Council of Techers (USA. 1989) have also identified five critical components that are characteristic of students who have good number sense:

number meaning
number relationships
number magnitude
operations
referents for numbers and quantities, i.e. numerals and variables

These components can be broadly split into prenumber concepts and number concepts.

Prenumber Concepts

Prenumber concepts involve those that provide a base for the understanding of quantities and how number systems work. These concepts develop understanding of number meaning, number relationships, and magnitude. These are generally developed using real-world physical objects.

Classifying

When students practice classifying, they are building understanding of different ways items can be grouped. We see classifying when children sort items by size, color, shape, or another quality.

Patterns

Recreating and continuing patterns helps students build pattern recognition. This patterning skill is essential in understanding and using number systems, which are based on patterns.

Comparisons

When playing with objects in groups, children can compare the sizes of the groups. Exploration of comparisons should include spatial (how closely or loosely packed are the items), dimensional (how big or small are the items in the group), and other attributes. Ultimately, students should arrive at an understanding of the concept of one-to-one comparison to determine which group is bigger or smaller, more or fewer (less).

Conservation

The concept of conservation is the understanding that something stays the same in quantity even though its appearance changes. To be more technical (but you don’t have to be) conservation is the ability to understand that redistributing material does not affect its mass, number or volume.

Subitizing

Subitizing is the ability to recognize the amount in a group quickly and without counting. When a student briefly sees the two rows of three pips on a die and recognizes it as 6, they are subitizing.

Number Concepts

Number concepts are those ideas and understandings that most non-mathmeticians think of as “math”. They are generally practiced using oral or written representations (numerals) of quantities. However, teachers should take care to always connect the symbolic representation (“four” or 4) to the number amount (* * * *).

Cardinality

Cardinality is the concept that, when counting objects, the last number counted in one-to-one counting is the amount. This reflects the understanding that a number names an amount. A student that has not yet grasped this concept will simply count the quantity over again when asked, “How many?”

Number Meaning

Number meaning is closely related to cardinality. Students should be able to recognize and explain that a numeral or “number” represents an actual amount. (Unfortunately, too many primary grade educators still use “number” when they should be referring to 98 or 7 + 4 as numerals.) When given a number, a student should be able to represent it using physical objects, illustrations, or even using tools such as number lines (as long as they understand that the numeral on the line represents all the steps taken from 0 to arrive at that point).

Referents for Numbers/Quantities

Referents are simply the symbols or glyphs we write to represent numbers. Most of the time this means numerals such as 1, 5, or 396, but it can also mean word forms like “two” and “a million”. Students should also understand that the same number can be represented in multiple ways, e.g. 42, 40 + 2, 6 × 7, etc. Exploring and using the various forms a number can take also builds understanding of place value and how the numeral represention is “built”. Finally, it leads to the understanding of variables like y and π being numbers not letters.

Number Magnitude

Magnitude is the concept of the “big-ness” of a number/amount, especially in relation to other numbers. Students should have multiple experiences with numbers on number lines and with objects and illustrations to be able to reasonably estimate random quantities. For example, does the collection of orange balls look like 30, 60, or 100? Students should also understand that magnitude scales may change. When comparing 5 and 10, one is twice as much as the other and can be easily shown to be so, but when comparing 72 and 77, they are very “close” on the classroom number line, while 6341 and 6346 can be considered almost “the same” in certain situations. Yet, all three pairs are separated by the same amount, 5.

Understanding of magnitude helps students check for reasonableness in their answers and make estimations by understanding how to round numbers and when it is useful and appropriate to do so.

Relationships Between Numbers

Students must learn to identify and use the relationships between numbers. This includes using rules and patterns as well as using the activities of composing (making a new number by putting other numbers together) and decomposing (breaking a number into two or more of its parts). Students should be able to do things such as recognize that in our base-10 system^‡, decades (multiples of 10) can be added to other numbers by counting up by ten. They should be able to group numbers based on attributes such as odd/even or prime/composite. Understanding these relationships helps build fluency and accuracy when students begin manipulating numbers through operations.

Operations Involving Numbers

Once students become fluent in identifying and using relationships between numbers, they will find traditional math operations easier to understand and complete. A problem such as 345 - 287 might be solved more easliy with a technique such as compensation by adding 13 to both numbers and subtracting 300. You can probably do it in your head! Instead of relying on paper and pencil and traditional algorithms, they will be able to flexibly apply their understanding to solve addition, subtraction, multiplication, and division equations. Strong number sense also leads to not only completing operations with whole numbers but also understanding and using partial numbers such as numbers using decimal points/places and fractions.

THE BEARS AND THE GRAPES Once upon a time, there were three bears. The bears were hungry, so each bear ate 2 apples. Then they were not hungry any more. How many apples did they eat?

Problem Solving

Problem solving (word or story problems, situational math) naturally derives from the previously mentioned number and prenumber concepts. However, an argument can be made that teachers should be having students explore and solve these types of problems even before students can write or recognize numeral representations. By interacting with language, students as young as 4 or 5 can solve simple problems using objects. They can even “do” multiplication. Using toy apples (or blocks or beans, etc.), they can make groups of two for each bear and count them all. This concrete manipulation exactly represents the expression 3 × 2, and understanding this relationship between the story problem and the number manipulation used to solve it is exactly the goal of developing number sense.

Measurement

Finally, measurement is another number aspect of number sense that is useful to develop and explore. It is related to the prenumber concept of comparison as well as the number concepts of magnitude and referents. When developing the idea and practice of measurement, students should be given opportunities to explore with various units of measure. This will lead them to the understanding that measurement is always an approximation because it can always be made more precise. When measuring a book with paper clips, you get a result (number and unit) that is close but maybe not exact, but if you measure using 1cm counting cubes you will get a result that is likely closer to the actual book length.

Conclusion

As early as 1994, the National Council of Teachers of Mathematics has been encouraging the development of number sense in students. Unfortunately, few school districts and education systems have prioritized this until very recently. There is much research into the benefits of explicitly developing number sense in our beginning and intermediate grade students. However, the wheels of beaurocracy are slow to turn and number sense "instruction” has just begun (as of 2014) to appear in some math textbooks, though not necessarily in those put out by the nation-wide publishers that perennially get the most school district contracts. Hopefully, you can use the activities and information presented here to begin to develop number sense in your child or students. If you are a parent or teacher, please pass along this document to your child's teacher or your colleagues, so we can begin to help even more children in their journey through mathematics!

^‡ - There are more number systems than our base-10 (decimal) system, and many are used throughout the sciences. Understanding number relationships also works in those systems! For example, in octal (base-8), decimal 12 is represented as “(14)₈”, one "eight” and four “ones”. Adding 8 to this number simply advances the “eights” place so it becomes “(24)₈” which translates to decimal 20. (2 in the eights place is 2 × 8, or decimal 16, which is then added to the 4 to make 20!) ↩

BIBLIOGRAPHY

“Activities to Help Your Child Develop Number Sense.” Fisherkindercorner, n.d. Web. 24 Oct. 2014. https://sites.google.com/site/fisherkindercorner/Home/ideas-to-help-your-child-develop-number-sense.
“Boosting Number Sense.” Scholastic.com. N.p., n.d. Web. 24 Oct. 2014. http://www.scholastic.com/parents/resources/article/what-to-expect-grade/boosting-number-sense.
Carpenter, T. P., Fennema, E., Franke, M. L., Levi, L., & Empson, S. B. “Cognitively Guided Instruction: A Research-Based Teacher Professional Development Program for Elementary School Mathematics.” National Center For Improving Student Learning & Achievement In Mathematics & Science. Sep. 2000. Web. 29 Oct. 2017. http://ncisla.wceruw.org/publications/reports/RR00-3.PDF
Connell, Mike. “Number Sense: What It Is, Why It’s Important, and How It Develops.” Native Brain. N.p., 27 Nov. 2012. Web. 24 Oct. 2014. http://www.nativebrain.com/2012/11/number-sense-what-it-is-why-its-important-and-how-it-develops/
Glasgow, Brennan. “Ways to Work on Number Sense.” Regional School District #10, n.d. Web. 24 Oct. 2014. http://www.region10ct.org/math/region10mathsitefaq/whtwaysworknmbersense.html.
Glasgow, Brennan. “What is Number Sense?” Regional School District #10, n.d. Web. 24 Oct. 2014. http://www.region10ct.org/math/region10mathsitefaq/whatisnumbersense.html.
Griffin, Sharon. “Teaching Number Sense” Educational Leadership vol. 61, no. 5, 2004. Web. 27 Oct. 2014. https://files.ascd.org/staticfiles/ascd/pdf/journals/ed_lead/el200402_griffin.pdf.
Kepner, Henry (Hank). “A Missed Opportunity: Mathematics in Early Childhood.” Editorial. NCTM Summing Up Feb. 2010: n. pag. NCTM.org. Web. 24 Oct. 2014. http://www.nctm.org/about/content.aspx?id=25054.
MacNee, Anne. “Activities Kids Can Do at Home to Develop Number Sense Handout2.” Scribd. N.p., n.d. Web. 24 Oct. 2014. https://www.scribd.com/doc/57759461/Activities-Kids-Can-Do-at-Home-to-Develop-Number-Sense-Handout2
McLeod, Saul. “Concrete Operational Stage.” Simply Psychology. N.p., n.d. Web. 29 Oct. 2014. http://www.simplypsychology.org/concrete-operational.html.
Moomaw, Sally. “Cardinality in the Early Learning and Development Standards.” Early Childhood Building Blocks (n.d.): n. pag. Resources for Early Childhood. Web. 3 Nov. 2014. http://rec.ohiorc.org/orc_documents/orc/recv2/briefs/pdf/0020.pdf
“Number Sense.” TeacherVision. N.p., n.d. Web. 24 Oct. 2014. https://www.teachervision.com/pro-dev/teaching-methods/48939.html.

Reys, Barbara J. “Promoting Number Sense in the Middle Grades” Mathematics Teaching in the Middle School vol. 1, no. 2, 1994. Web. 13 Nov. 2014. https://pubs.nctm.org/view/journals/mtms/1/2/article-p114.xml.
Way, Jenni. “Number Sense Series: Developing Early Number Sense.” NRICH. 2005. Web. 2 Nov. 2014. https://nrich.maths.org/2477.

Originally written in StackEdit.